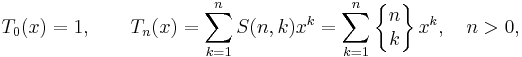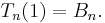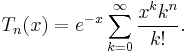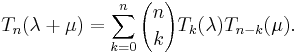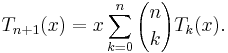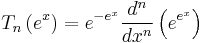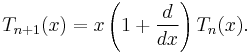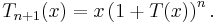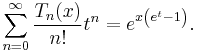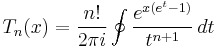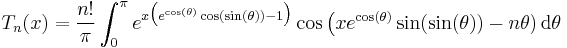Touchard polynomials

For a different family of polynomials Qn occasionally called Touchard polynomials, see Bateman polynomials.

The Touchard polynomials, studied by Jacques Touchard (1939), also called the exponential polynomials, comprise a polynomial sequence of binomial type defined by

T_0(x) = 1,\qquad T_n(x)=\sum_{k=1}^n S(n,k)x^k=\sum_{k=1}^n \left\{\begin{matrix} n \\ k \end{matrix}\right\}x^k, \quad n > 0,

where S(n, k) is a Stirling number of the second kind, i.e., it is the number of partitions of a set of size n into k disjoint non-empty subsets. (The second notation above, with { braces }, was introduced by Donald Knuth.) The value at 1 of the nth Touchard polynomial is the nth Bell number, i.e., the number of partitions of a set of size n:

T_n(1)=B_n.

If X is a random variable with a Poisson distribution with expected value λ, then its nth moment is E(Xn) = Tn(λ), leading to the definition:

T_{n}(x)=e^{-x}\sum_{k=0}^\infty \frac {x^k k^n} {k!}.

Using this fact one can quickly prove that this polynomial sequence is of binomial type, i.e., it satisfies the sequence of identities:

T_n(\lambda%2B\mu)=\sum_{k=0}^n {n \choose k} T_k(\lambda) T_{n-k}(\mu).

The Touchard polynomials make up the only polynomial sequence of binomial type in which the coefficient of the 1st-degree term of every polynomial is 1.

T_{n%2B1}(x)=x\sum_{k=0}^n{n \choose k}T_k(x).

The Touchard polynomials satisfy the Rodrigues-like formula:

T_n \left(e^x \right) = e^{-e^x} \frac{d^n}{dx^n}\left(e^{e^x}\right)

The Touchard polynomials satisfy the recursion

T_{n%2B1}(x)=x \left(1%2B\frac{d}{dx} \right)T_{n}(x).

And

T_{n%2B1}(x)=x\sum_{k=0}^n{n \choose k}T_k(x).

In case x = 1, this reduces to the recursion formula for the Bell numbers.

Using the Umbral notation Tn(x)=Tn(x),these formulas become:

T_n(\lambda%2B\mu)=\left(T(\lambda)%2BT(\mu) \right)^n .
T_{n%2B1}(x)=x \left(1%2BT(x) \right)^n.

The generating function of the Touchard polynomials is

\sum_{n=0}^\infty {T_n(x) \over n!} t^n=e^{x\left(e^t-1\right)}.

And a contour-integral representation is

T_n(x)=\frac{n!}{2\pi i}\oint\frac{e^{x({e^t}-1)}}{t^{n%2B1}}\,dt

The Touchard polynomials (and thereby the Bell numbers) can be generalized, using the real part of the above integral, to non-integer order:

T_n(x)=\frac{n!}{\pi} \int^{\pi}_0 e^{x \bigl(e^{\cos(\theta)} \cos(\sin(\theta))-1 \bigr)} \cos \bigl(x e^{\cos(\theta)} \sin(\sin(\theta)) -n\theta) \, \mathrm{d}\theta

References